

Thus, it makes sense to consider the values of ∫ a t f ( x ) d x ∫ a t f ( x ) d x as t t approaches b b for a < t < b. Consider an integral of the form ∫ a b f ( x ) d x, ∫ a b f ( x ) d x, where f ( x ) f ( x ) is continuous over for all values of t t satisfying a < t < b, a < t < b, the integral ∫ a t f ( x ) d x ∫ a t f ( x ) d x is defined for all such values of t. Now let’s examine integrals of functions containing an infinite discontinuity in the interval over which the integration occurs. State whether the improper integral converges or diverges. In other words, we may define an improper integral as a limit, taken as one of the limits of integration increases or decreases without bound.Įvaluate ∫ −3 + ∞ e − x d x. Figure 3.17 shows that ∫ a t f ( x ) d x ∫ a t f ( x ) d x may be interpreted as area for various values of t. How should we go about defining an integral of the type ∫ a + ∞ f ( x ) d x ? ∫ a + ∞ f ( x ) d x ? We can integrate ∫ a t f ( x ) d x ∫ a t f ( x ) d x for any value of t, t, so it is reasonable to look at the behavior of this integral as we substitute larger values of t. We examine several techniques for evaluating improper integrals, all of which involve taking limits. Integrals of these types are called improper integrals.
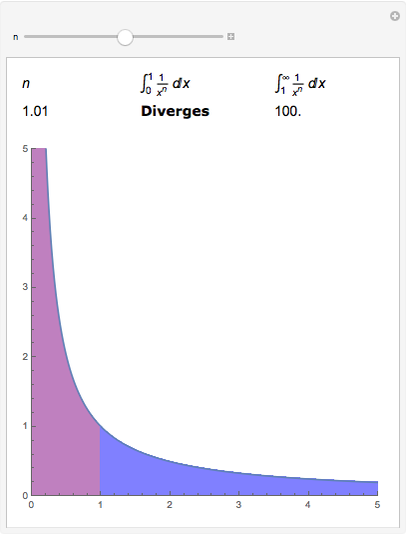
In this section, we define integrals over an infinite interval as well as integrals of functions containing a discontinuity on the interval. Is the area between the graph of f ( x ) = 1 x f ( x ) = 1 x and the x-axis over the interval [ 1, + ∞ ) [ 1, + ∞ ) finite or infinite? If this same region is revolved about the x-axis, is the volume finite or infinite? Surprisingly, the area of the region described is infinite, but the volume of the solid obtained by revolving this region about the x-axis is finite. 3.7.3 Use the comparison theorem to determine whether a definite integral is convergent.3.7.2 Evaluate an integral over a closed interval with an infinite discontinuity within the interval.3.7.1 Evaluate an integral over an infinite interval.


 0 kommentar(er)
0 kommentar(er)
